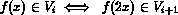
The scaling property
and the fact that {
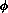 (t-j): j
(t-j): jis an orthogonal basis for Vi. EXERCISE: show this.
In general, for any function g(x) and j,k![]()
![]() ,
we define
,
we define
Then the above shows that {
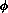 j,k: k
j,k: kRestricting attention to V1, this means
for some ck's. This is called the dilation equation. We can of course obtain ck as the projection of
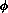 onto sqrt(2)
onto sqrt(2)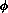 (2x-k),
(2x-k),
Let us now derive several properties of the ck's.
In proof, use the fact that
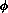 (x)
(x)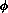 (x-m)dt=
(x-m)dt=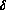 (m),
and substitute the dilation equation for
(m),
and substitute the dilation equation for 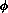 (x) and for
(x) and for
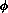 (x-m).
(x-m).
Proof sketch: use the fact that
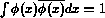 and substitute the dilation equation for
and substitute the dilation equation for 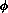 (x).
(x).
where
where we write hk for 2-1/2ck. In proof, we just take the Fourier transform of both sides of the dilation equation to give us
The transformation of this infinite sum to the above formula () actually require justification (see [3], p.54).
Iterating this, we obtain
Note that
by the normalization assumption
We may also assume
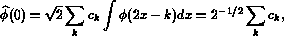 . Hence
. Hence
This infinite product is defined if H(0)=1. Even if H(0)
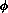 (t) to the dilation
equation. [Show]
(t) to the dilation
equation. [Show]
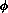 (t). It is easy
to see from this iteration that the solution
(t). It is easy
to see from this iteration that the solution 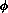 (t)
is supported in the interval [N,-N].
(t)
is supported in the interval [N,-N].